IM Geometry Unit 3 Lesson 8

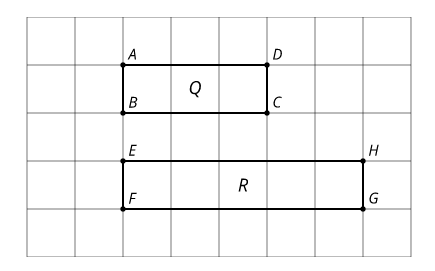
Are these rectangles similar? Explain how you know.

Tyler wrote a proof that all rectangles are similar. Make the image Tyler describes in each step in his proof. Which step makes a false assumption? Why is it false? 1. Draw 2 rectangles. Label on ABCD and the other PQRS. 2. Translate rectangle ABCD by the directed line segment from A to P. A' and P now coincide. 3. Rotate rectangle A'B'C'D' by angle D'A'S. Segment A"D" now lies on ray PS. 4. Dilate rectangle A"B"C"D" using center A" and scale factor . 5. Because all angles of a rectangle are right angles, segment A'''B''' now lies on ray PQ. (If A'''B''' and PQ don't coincide, reflect across PS. 6. Dilate rectangle A'''B'''C'''D''' using center A''' and scale factor . 7. Due to the symmetry of a rectangle, if 2 rectangles coincide on 2 sides, they must coincide on all sides

Choose one statement from the list. Decide if it is true or not. If it is true, write a proof. If it is not true, provide a counterexample

1. All equilateral triangles are similar.
2. All isosceles triangles are similar.
3. All right triangles are similar.
4. All circles are similar.