Silhouettes without gaps and isolated
This activity belongs to the GeoGebra book Voronoi Paintings.
Indeed, we aim to maximize the contrast between these two spaces, positive and negative, so we will consider positive space as consisting of focal areas that are gap-free and isolated.
If a focal area has a "gap", meaning it includes an internal color spot that we would interpret as negative space, we will treat that gap as an integral part of the positive space.
For example, in Figure 7 we see Van Gogh's painting Sunflowers. To its right, in black, is its positive space, the silhouette of the vase with flowers. Notice how the gaps, the spaces between flowers, have been removed to create a gap-free form.
Figure 7: Gap-free silhouette of a focal area
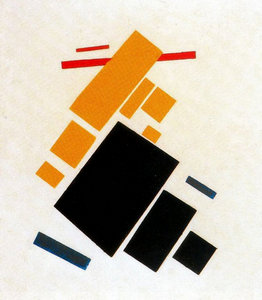
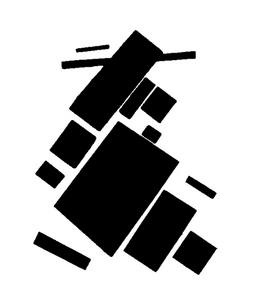
Figure 8: Two focal areas merging into a single silhouette
Authors of the activity: Rafael Losada & Tomás Recio.