Google Classroom
GeoGebraGeoGebra Classroom
GeoGebra

Similarity

Author:Christopher Rose

Transformations

Graph △FGH with vertices F(−2, 2), G(−2,−4) and H(−4,−4) and its image after the similarity transformation. Translation: (x, y) → (x+3, y+1) Dilation: (x, y) → (2x, 2y)
Graph △FGH with vertices F(−2, 2), G(−2,−4) and H(−4,−4) and its image after the similarity transformation. Rotation:  90° about the origin Dilation: (x, y) → (3x, 3y)

Describe at least one similarity transformation that maps the blue preimage to the pink image. Include any transformational rules that are used in your process. (example: if you have a translation right three and up one and a half scale dilation, you would include (x+3, y+1) and (.5x, .5y) in your answer)

Describe at least one similarity transformation that maps the blue preimage to the pink image. Include any transformational rules that are used in your process. (example: if you have a translation right three and up one and a half scale dilation, you would include (x+3, y+1) and (.5x, .5y) in your answer)

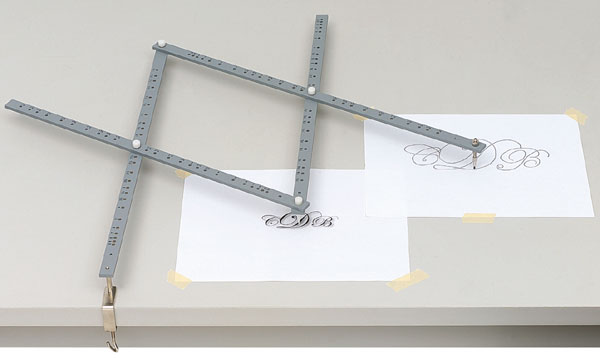
This is a picture of an artist's pantograph. There are many kinds of pantographs. For example, cartographers, carpenters, and architects all sometimes use versions of this tool.
This is a picture of an artist's pantograph. There are many kinds of pantographs. For example, cartographers, carpenters, and architects all sometimes use versions of this tool.

In the picture given of a pantograph. The distance from the tracing element is one half the distance from the mounting point as the drawing element. This makes a dilation with a scale factor of two. What would happen if the tracing element was set up at a distance of 3 inches and the drawing element was set to 7 inches? Be as specific and precise as you can.

New Resources

Discover Resources

Discover Topics