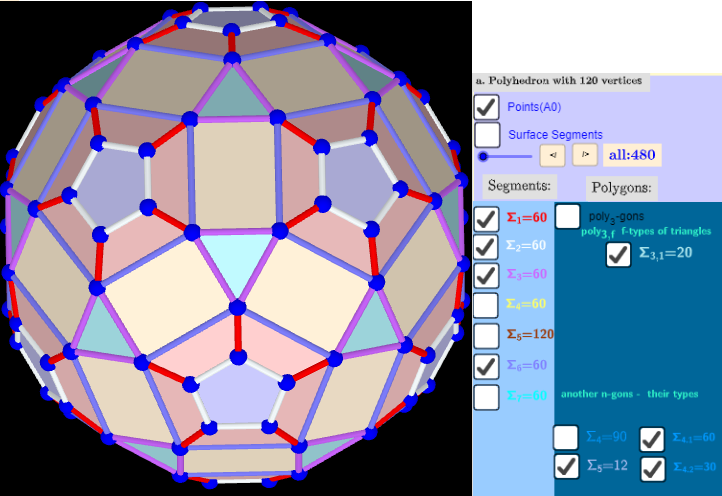
Rhombicosidodecahedron from Biscribed Pentakis Dodecahedron for the case of trisection of its 1st-order segments
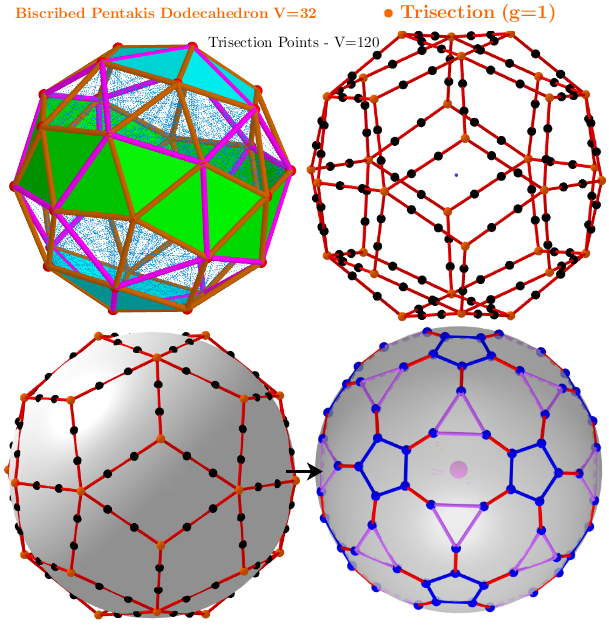
A polyhedron is constructed whose V=120 vertices are the points of the trisection of the segments the same length 1st-order (g=1) of the Biscribed Pentakis Dodecahedron.
Geometric Constructions are in Applet: Series of polyhedra obtained by trisection (truncation) different segments of the original polyhedron, and the resulting polyhedra in Applet: Serie of polyhedra obtained by trisection (truncation) segments of the Biscribed Pentakis Dodecahedron.

1. Generating Elements of mesh modeling the surfaces of convex polyhedron and its dual image
2. Coloring edges and faces of polyhedra
If we assume that all quadrilaterals lie in the same plane, then our polyhedron approximately looks like
Rhombicosidodecahedron :
https://robertlovespi.net/2014/06/02/zonish-versions-of-the-rhombicosidodecahedron/
This polyhedron based on adding zones along the five-fold symmetry axes of a rhombicosidodecahedron.
All its edges are the same length, and its
62 faces include
20 triangles,
12 regular pentagons and
30 elongated octagons.
Properties of polyhedra
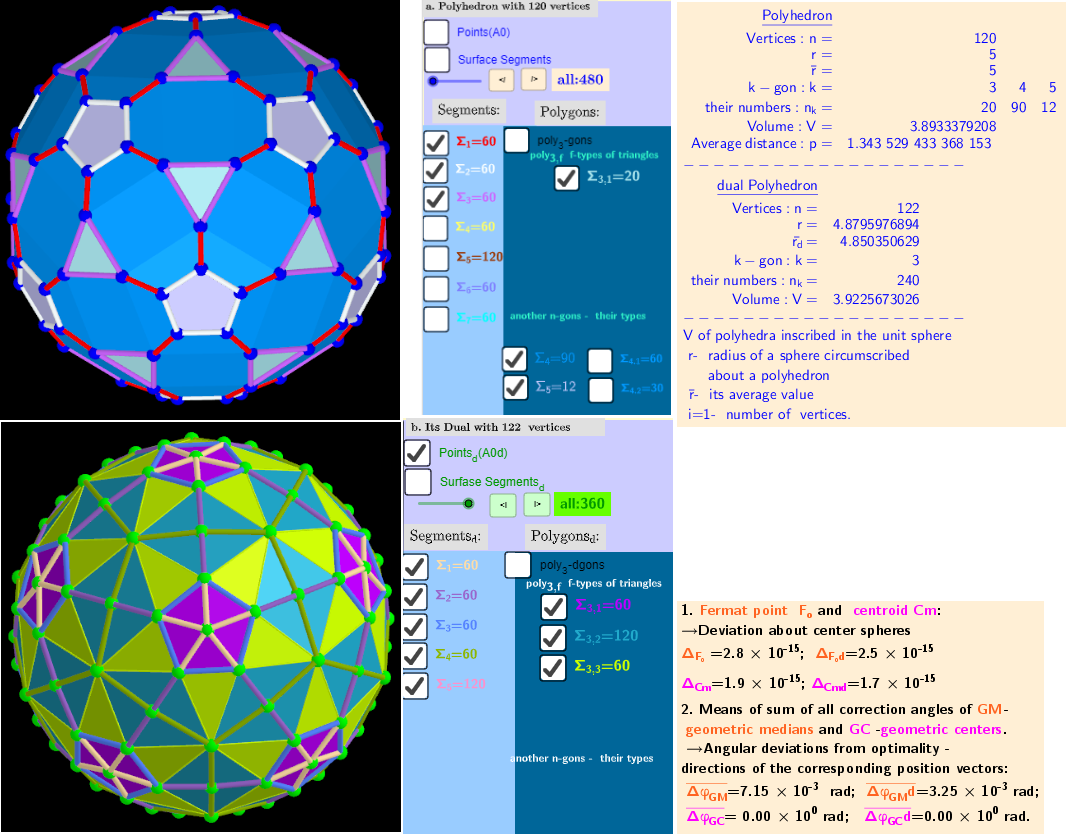
Actual location of the quadrilaterals