Banked Track with Friction
In virtually all cases when vehicles like race cars go around turns that are banked, there will be friction involved. The solution of this problem is slightly more challenging than most friction problems we've solved in the past because the value of friction may not be written as a simple multiple of the normal force. The reason why is that it is very likely that friction will not be at its limit, which as you recall is what we mean when we write That's why we were careful to write that equation as an inequality for non-slipping cases. (Once slipping friction does its best to end the slipping.)
The other difficulty with friction in this problem is that it affects the normal force. So the normal force allows friction to be generated and once generated, the normal force changes.
The best way to deal with this problem is to leave friction written as friction force rather than expressing in terms of normal force until all the work is done. Then you can test with numbers if a given scenario is possible based on the fact that
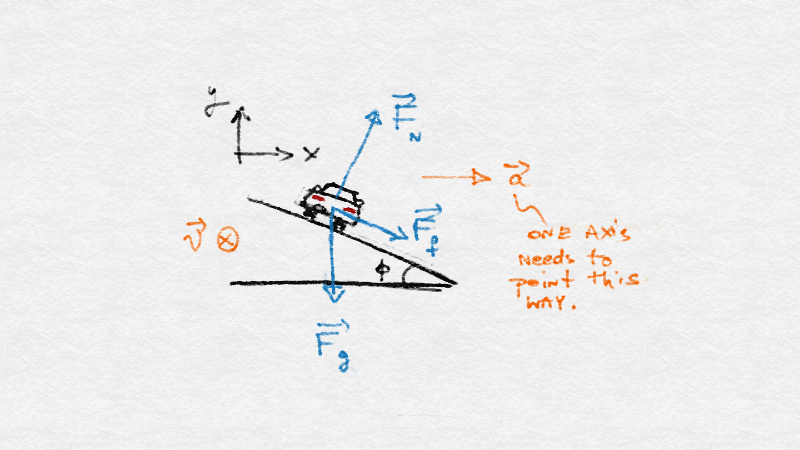
The Solution
Using the coordinates shown in the diagram, we get the following two equations:
As is always true, you can only solve for two unknowns with two equations. Suppose we want to know the normal force and the friction force. Doing the algebra is messier than the frictionless case, but that's just a fact of life.
Since you know how to do algebra, rather than showing you how to solve it by hand, I'll use this as an opportunity to show you the GeoGebra computer algebra system (CAS). It is a view within GeoGebra that allows symbolic solution of math equations. I'll solve these two equations first symbolically, and then will use that system to plug in values of m=1200kg, v=20m/s, g=9.8m/s2, r=60m, and
Besides literally copying the input below into your own GeoGebra session, you need to know that the last line will not evaluate to a decimal unless you highlight cell 4 and click the 
icon. This allows an approximate numerical representation of irrational numbers.
icon. This allows an approximate numerical representation of irrational numbers.GeoGebra CAS Example
Looking at the results, we see that the friction force is 4167N and the normal force is 13,599N. So we should ask whether the car would be able to round such a corner. We need to be sure that the coefficient of friction is sufficient to allow this. Since we can, for instance solve for the coefficient necessary to round the corner. Algebraically, this gives So long as the car isn't rounding an icy corner, it'll probably be fine since even economy car tires on dry pavement have friction coefficients of at least 0.7 and race tires can exceed 1.1.