Google Classroom
GeoGebraGeoGebra Classroom
GeoGebra

Lesson 1; Number Puzzles

Author:Arpit Kesharwani

Warm Up

What do you notice? What do you wonder?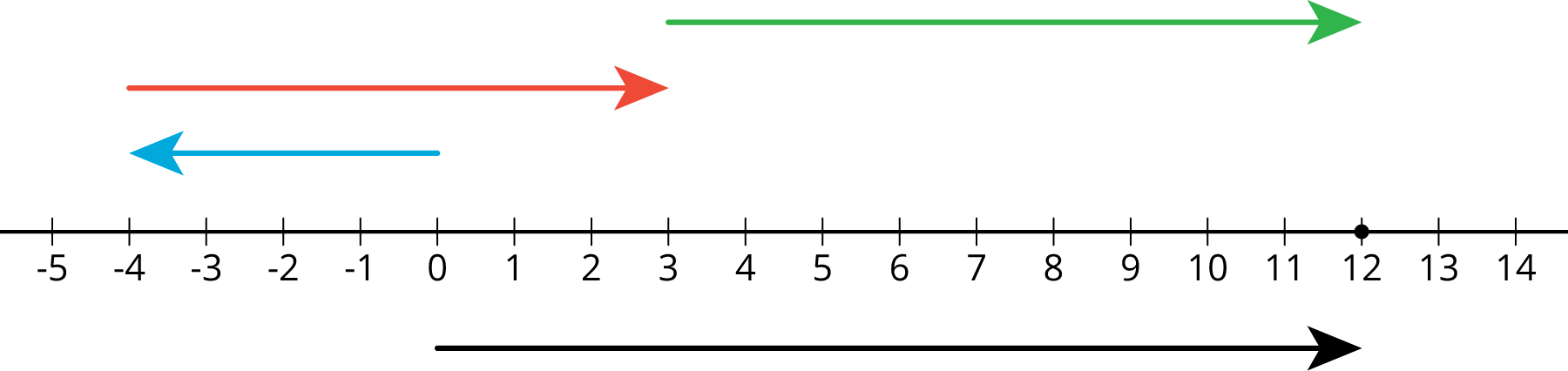

Check my answer
Activity Synthesis After students’ notice and wonder ideas are displayed, tell them that the diagram is about money, and invite students to share a possible story and equation that the diagram represents. For example, someone owes $4. Then they earn $7 doing chores and another $9 helping out the neighbor with their yard. The equation would be -4+7+9=12.

Recap of Linear Equations; OPTIONAL Video

Activity 1

In this task, students create their own number puzzle to trade with a partner to solve. The purpose of this task is for students to practice writing and solving multi-step number puzzles and compare their representations with the representations of others to decide which are more efficient. While these problems are phrased using the words “number puzzle,” it is important to note the mathematical work students are doing here thinking about, creating, and solving situations that are, essentially, linear equations in one variable, even if not all students are using equations to represent them.While students are sharing representations, identify partners who solved at least one of their puzzles using different representations to share during the whole-class discussion. If possible, select groups who used an equation. Launch Keep students in the same groups. Give 5 minutes for students to write their own puzzle and make a representation of their solution before trading their puzzle with a partner to solve. Make sure students write their puzzle and solution in such a way that when they trade, their partner cannot see the solution.If both partners created the same (or very similar) representation for their solutions, ask them to work together to create a different representation. If they created different representations, ask partners to discuss which one they prefer and to be ready to explain why during the whole-class discussion.

Assignment

Student-Facing Task StatementWrite another number puzzle with at least three steps. On a different piece of paper, write a solution to your puzzle.Trade puzzles with your partner and solve theirs. Make sure to show your thinking.With your partner, compare your solutions to each puzzle. Did they solve them the same way you did? Be prepared to share with the class which solution strategy you like best.

Check my answer

Are you Ready for More?

Here is a number puzzle that uses math. Some might call it a magic trick!

  1. Think of a number.
  2. Double the number.
  3. Add 9.
  4. Subtract 3.
  5. Divide by 2.
  6. Subtract the number you started with.
  7. The answer should be 3.
Why does this always work? Can you think of a different number puzzle that uses math (like this one) that will always result in 5?

Check my answer

Teacher's Summary/Note

Activity Synthesis Select partners previously identified to share a puzzle with the class and the two representations they created. Ask which representation they prefer and why. If students do not bring it up in their explanations, ask which of their representations was the most efficient one for solving the puzzle.During this discussion, students may ask you to state which representation is best and, if so, it is important to note that there is no one correct answer for the “best representation.” The “best representation” is the one that makes sense to the student and helps them solve the problem. However, as problems grow more and more complex, students are likely to find that certain representations are more useful for solving problems than others. Lesson Synthesis Ask students to think about what their number line diagrams, tape diagrams, and equations represented in each of the activities. Guide them in seeing that stories with an unknown quantity usually involve actions, like the temperature rising, earning money by doing chores, or relationships, like Diego’s distance being half of Lin’s distance and 300 m more than Jada’s. Ask them to think about the puzzle they wrote in the last activity and whether they described actions or relationships. Invite their opinions about which representations best represent actions and which best represent relationships.Tell students to think about the expression x+5. Ask: “What could this mean in terms of two numbers being related to each other? What could this represent as an action?” (One number is 5 more than the other. A sample action: the temperature increases by 5 degrees.)If time allows, ask students to make up another puzzle that describes actions if they previously chose relationships, and vice versa, and to represent their puzzles with a diagram and an equation. Display diagrams and equations for all to see.

Exit Ticket/Extra Practice ~ OPTIONAL

Andre and Elena are reading the same book over the summer. Andre says he has read 15 of the book. Elena says she has read 20 more pages than Andre. If Elena is on page 55, how many pages are in the book?Lin has drawn a diagram to solve this question. Find her error.

Check my answer

New Resources

Discover Resources

Discover Topics