Introduction to Dilations
1.
Determine the scale factor and the location of your center of dilation from triangle ABC to triangle A'B'C'. Adjust your scale factor, "k" using the slider, and adjust the location of the center of dilation, "P" until you have mapped triangle ABC on to triangle A'B'C'.
2.
Determine the scale factor and the location of your center of dilation from polygon ABCDE to polygon A'B'C'D'E'. Adjust the scale factor, "k" using the slider, and adjust the location of the center of dilation, "P" until you have mapped polygon ABCDE on to polygon A'B'C'D'E'.
3.
Determine the scale factor and the location of your center of dilation from quadrilateral ABCD to quadrilateral A'B'C'D'. Adjust the scale factor, "k" using the slider, and adjust the location of the center of dilation, "P" until you have mapped triangle ABC on to triangle A'B'C'.
4.
Determine the scale factor and the location of your center of dilation from triangle ABC to triangle DEF. Adjust the scale factor, "k" using the slider, and adjust the location of the center of dilation, "P" until you have mapped triangle ABC on to triangle DEF.
Use your discoveries from examples 1-4 to answer the following questions.
How does the center of dilation affect how a shape "dilates"? What happens when the point is away from the shape, in the middle of the shape, or on a vertex?
When the scale factor is greater than one, does the image move closer to the center of dilation or further away?
When the scale factor is less than one, does the image move closer to the center of dilation or further away?
Why were we unable to map triangle ABC on to triangle DEF in question 4?
5.
The scale factor from triangle ABC to triangle A'B'C' is equal to 1/3. Determine the location of the center of dilation. Use the ray tool 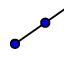 to connect corresponding points starting with the larger image, then use the point tool
to connect corresponding points starting with the larger image, then use the point tool to mark where the rays intersect. Since our scale factor is less than one, our image is smaller then our preimage, so you must draw your ray starting with one preimage point and then selecting the corresponding image point. For example, select the ray tool,
to mark where the rays intersect. Since our scale factor is less than one, our image is smaller then our preimage, so you must draw your ray starting with one preimage point and then selecting the corresponding image point. For example, select the ray tool, 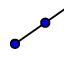 , then select A, and then select A'.
, then select A, and then select A'.
 to connect corresponding points starting with the larger image, then use the point tool
to connect corresponding points starting with the larger image, then use the point tool to mark where the rays intersect. Since our scale factor is less than one, our image is smaller then our preimage, so you must draw your ray starting with one preimage point and then selecting the corresponding image point. For example, select the ray tool,
to mark where the rays intersect. Since our scale factor is less than one, our image is smaller then our preimage, so you must draw your ray starting with one preimage point and then selecting the corresponding image point. For example, select the ray tool,  , then select A, and then select A'.
, then select A, and then select A'. 6.
The scale factor from quadrilateral ABCD to quadrilateral A'B'C'D is equal to 2.5. Determine the location of the center of dilation. Use the ray tool  to connect two pairs of corresponding points starting with the larger image, then use the point tool
to connect two pairs of corresponding points starting with the larger image, then use the point tool to mark where the rays intersect. Since our scale factor is greater than one, our image is larger then our preimage, so you must draw your ray starting with one image point and then selecting the corresponding preimage point. For example, select the ray tool,
to mark where the rays intersect. Since our scale factor is greater than one, our image is larger then our preimage, so you must draw your ray starting with one image point and then selecting the corresponding preimage point. For example, select the ray tool,  , then select A', and then select A.
, then select A', and then select A.
 to connect two pairs of corresponding points starting with the larger image, then use the point tool
to connect two pairs of corresponding points starting with the larger image, then use the point tool to mark where the rays intersect. Since our scale factor is greater than one, our image is larger then our preimage, so you must draw your ray starting with one image point and then selecting the corresponding preimage point. For example, select the ray tool,
to mark where the rays intersect. Since our scale factor is greater than one, our image is larger then our preimage, so you must draw your ray starting with one image point and then selecting the corresponding preimage point. For example, select the ray tool,  , then select A', and then select A.
, then select A', and then select A. 7
Determine the scale factor from triangle ABC to triangle A'B'C'. Measure the distance from A to B by selecting the distance tool  , then select each point. Measure the distance from A' to B'.
Measure the distance from P to B by first selecting the distance tool
, then select each point. Measure the distance from A' to B'.
Measure the distance from P to B by first selecting the distance tool  , then click on P followed by B. Repeat this procedure to measure the distance from P to B'.
Select the angle measure tool, and measure angle BAC and angle B'A'C'. Measure all other pairs of corresponding angles
, then click on P followed by B. Repeat this procedure to measure the distance from P to B'.
Select the angle measure tool, and measure angle BAC and angle B'A'C'. Measure all other pairs of corresponding angles
 , then select each point. Measure the distance from A' to B'.
Measure the distance from P to B by first selecting the distance tool
, then select each point. Measure the distance from A' to B'.
Measure the distance from P to B by first selecting the distance tool  , then click on P followed by B. Repeat this procedure to measure the distance from P to B'.
Select the angle measure tool, and measure angle BAC and angle B'A'C'. Measure all other pairs of corresponding angles
, then click on P followed by B. Repeat this procedure to measure the distance from P to B'.
Select the angle measure tool, and measure angle BAC and angle B'A'C'. Measure all other pairs of corresponding anglesUse your measurements from example 7 to answer the following questions.
Set up a ratio of side A'B' divided by side AB. What is the scale factor from triangle ABC to triangle A'B'C?
Set up a ratio of P'B' divided by PB. Does this ratio match your scale factor?
How do corresponding angles compare under a dilation?
8a.
Determine the dilation that takes triangle ABC to triangle A'B'C'. Find your scale factor and center of dilation. Then, scroll down to 8b, and follow the appropriate directions
8b.
Using the scale factor and center of dilation found in 8a, plot your center of dilation using the point tool  and then dilate triangle ABC. Select the dilate from point tool
and then dilate triangle ABC. Select the dilate from point tool 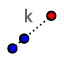 , click on the inside of triangle ABC, then type in your scale factor. Check to make sure your dilated image matches triangle A'B'C' in 8a.
, click on the inside of triangle ABC, then type in your scale factor. Check to make sure your dilated image matches triangle A'B'C' in 8a.
 and then dilate triangle ABC. Select the dilate from point tool
and then dilate triangle ABC. Select the dilate from point tool  , click on the inside of triangle ABC, then type in your scale factor. Check to make sure your dilated image matches triangle A'B'C' in 8a.
, click on the inside of triangle ABC, then type in your scale factor. Check to make sure your dilated image matches triangle A'B'C' in 8a. 9a.
Determine the dilation that takes quadrilateral ABCD to quadrilateral A'B'C'D'. Find your scale factor and center of dilation. Then, scroll down to 9b, and and follow the appropriate directions
9b.
Using the scale factor and center of dilation found in 9a, plot your center of dilation using the point tool  and then dilate quadrilateral ABCD. Select the dilate from point tool
and then dilate quadrilateral ABCD. Select the dilate from point tool  , click on the inside of quadrilateral ABCD, then type in your scale factor. Check to make sure your dilated image matches quadrilateral A'B'C'D' in 9a.
, click on the inside of quadrilateral ABCD, then type in your scale factor. Check to make sure your dilated image matches quadrilateral A'B'C'D' in 9a.
 and then dilate quadrilateral ABCD. Select the dilate from point tool
and then dilate quadrilateral ABCD. Select the dilate from point tool  , click on the inside of quadrilateral ABCD, then type in your scale factor. Check to make sure your dilated image matches quadrilateral A'B'C'D' in 9a.
, click on the inside of quadrilateral ABCD, then type in your scale factor. Check to make sure your dilated image matches quadrilateral A'B'C'D' in 9a. 10. Just for Fun, creating a dynamic dilation
In order to create a dilation that is dynamic like questions 1-4, follow the directions below. You still have a limited tool pallet; however you have access to all of the menus.
Create your own polygon using the polygon tool 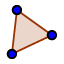 . Then, using the point tool
. Then, using the point tool  , plot a point away from your polygon; this will serve as your center of dilation.
Select dilate from point tool, and when the dialogue box pops up for you to input your scale factor, type in the letter k instead of a number. You should see an option pop up that says "Create a slider for k"? Click "create sliders."
You will now see a slider up above. I recommend right clicking on it, and under "slider" adjust the minimum to zero (a negative dilation ends up inverting the image).
There are a whole host of menus to access simply by right clicking on various objects. Simple things such as adjusting the colors are fun to play with.
, plot a point away from your polygon; this will serve as your center of dilation.
Select dilate from point tool, and when the dialogue box pops up for you to input your scale factor, type in the letter k instead of a number. You should see an option pop up that says "Create a slider for k"? Click "create sliders."
You will now see a slider up above. I recommend right clicking on it, and under "slider" adjust the minimum to zero (a negative dilation ends up inverting the image).
There are a whole host of menus to access simply by right clicking on various objects. Simple things such as adjusting the colors are fun to play with.
 . Then, using the point tool
. Then, using the point tool  , plot a point away from your polygon; this will serve as your center of dilation.
Select dilate from point tool, and when the dialogue box pops up for you to input your scale factor, type in the letter k instead of a number. You should see an option pop up that says "Create a slider for k"? Click "create sliders."
You will now see a slider up above. I recommend right clicking on it, and under "slider" adjust the minimum to zero (a negative dilation ends up inverting the image).
There are a whole host of menus to access simply by right clicking on various objects. Simple things such as adjusting the colors are fun to play with.
, plot a point away from your polygon; this will serve as your center of dilation.
Select dilate from point tool, and when the dialogue box pops up for you to input your scale factor, type in the letter k instead of a number. You should see an option pop up that says "Create a slider for k"? Click "create sliders."
You will now see a slider up above. I recommend right clicking on it, and under "slider" adjust the minimum to zero (a negative dilation ends up inverting the image).
There are a whole host of menus to access simply by right clicking on various objects. Simple things such as adjusting the colors are fun to play with.