The Catenary
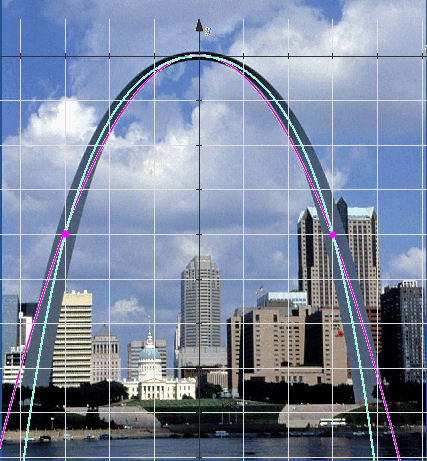
When introducing parabolas in high school math classes, many textbooks will use a picture of the Gateway Arch in St. Louis. Although similar to a parabola, the Gateway Arch was actually made to model a catenary. A catenary curve can be expressed as . They both happen to produce very similarly shaped curves, but they're not identical. There exists no parabola that would perfectly overlap with a catenary.
A parabola describes the motion of a projectile thrown into the air, disregarding air resistance.
A catenary is the shape of a cord suspended between two points. Gravity acts on the cord, pulling it down, but there is also tension in the cord, which acts along the cord.
We can define points O and P on the chain, just as we did with the bridge cable. We can even use the same free body diagram, and we get the same slope formula: (slope at P) = W/T. The trouble starts when we try to compute the weight, W. Unlike the bridge cable, the chain carries only its own weight. That weight is proportional to the length of the chain between O and P. It cannot be computed by simply multiplying the horizontal distance by a constant. We need some way to find the length of the chain between O and P.
As before, let the low point of the chain be the origin. Let y be the height of the chain. Again, μ will represent linear weight density. This time it is weight per length of chain. Let s be the length of the chain between O and P. This means that the weight of the chain between O and P must be μs.
You may recall this formula for the length of a curve on the interval (a, b):
curve length =
We can use it to find s, the curve length on the interval (0, x):
s=
The derivative, dy/dx, is itself a function of s.
Make this substitution for s in the integration equation above.
Differentiate both sides with respect to x.
We need to define y as a function of x, so that it satisfies this equation and the condition y(0) = 0. See if this one fits:
And the hyperbolic cosine is defined by this formula:
Even if you have never worked with hyperbolic trigonometry, you can apply elementary differential calculus skills to find the first and second derivatives of the function.
This solution was not actually derived here but there is enough information to verify it. One question remains unanswered. Why did Galileo believe that the catenary was a parabola? Mathematics aside, it would have been quite simple to test it experimentally. Plot a parabola and try to make a hanging chain fit the curve. It will not work. Try it.