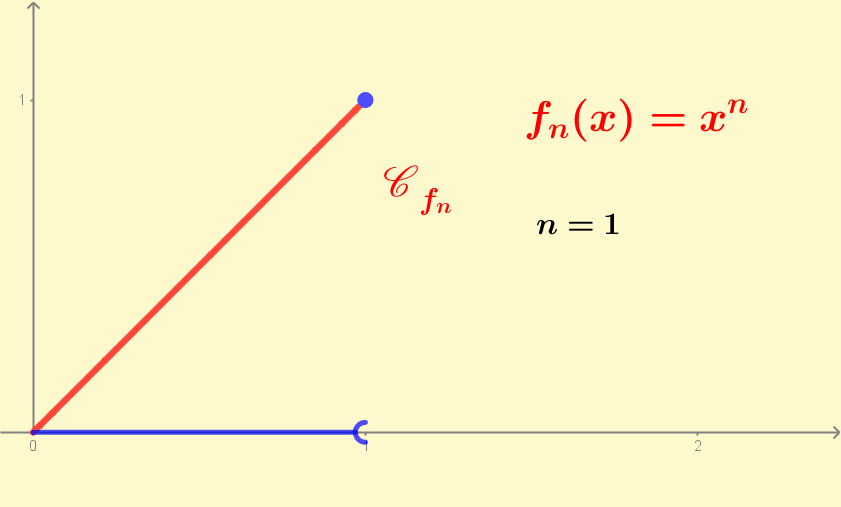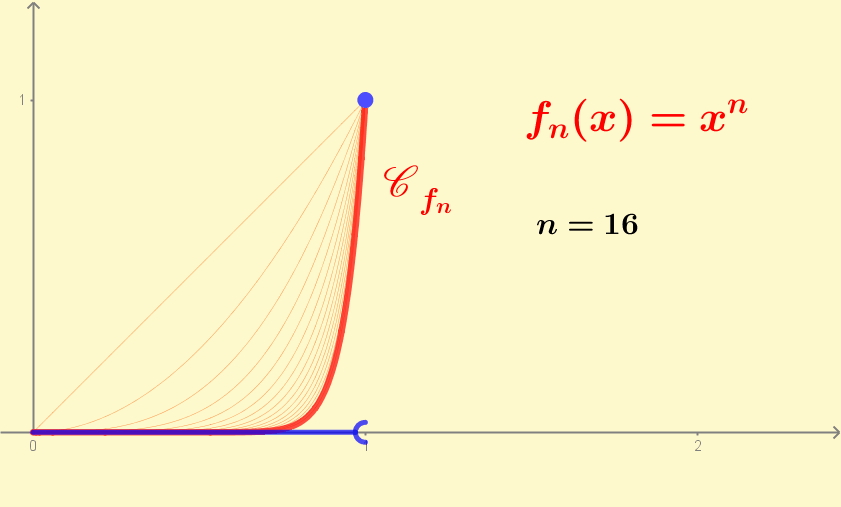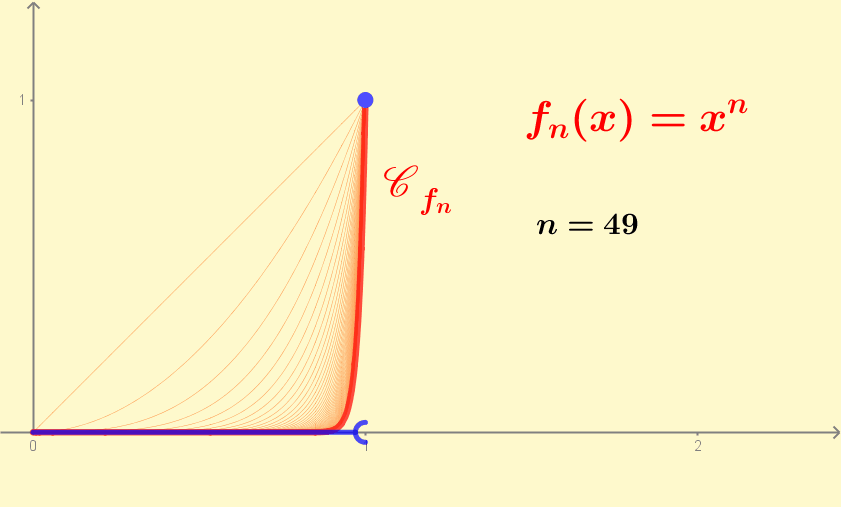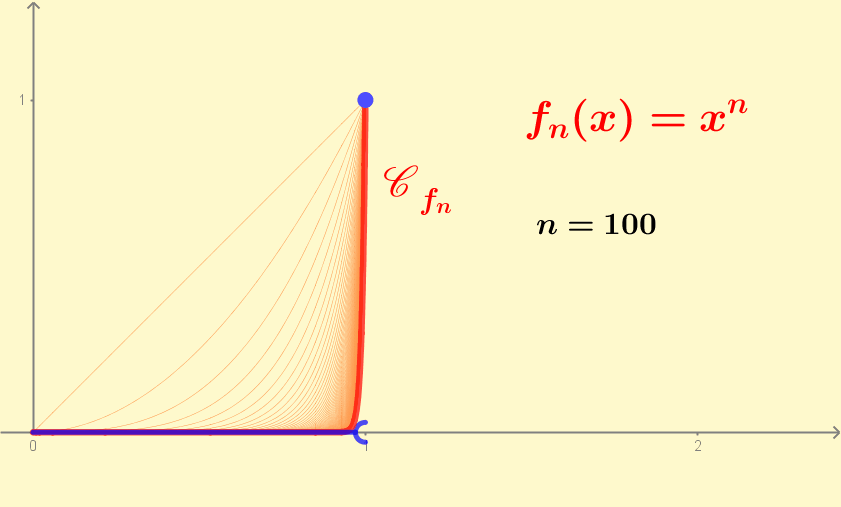Continuity is not preserved by pointwise convergence.
Here, the sequence of functions f_n (in red) converges pointwise to the function g (shown in blue). This illustrates that a sequence of continuous functions converging pointwise to a limiting function does not guarantee that the limit is also a continuous function. You can see that the limiting function g (in blue) has a discontinuity in x=1.
Uniform convergence on the other hand will provide a continuous limiting function.
Animated GIF