Investigative circle activity
Construct a perpendicular bisector of a line segment AB
Using the "segment command" 
, create a segment between two points A and B.
Choose th "midpoint or center tool" 
and click on the segment to get its midpoint
select the "perpendicular line tool" 
and click first on the midpoint and then on the segment.
You have got the perpendicular bisector of the line segment AB.
, create a segment between two points A and B.
Choose th "midpoint or center tool" and click on the segment to get its midpoint
select the "perpendicular line tool" and click first on the midpoint and then on the segment.
You have got the perpendicular bisector of the line segment AB.Let's investigate
Pick a point on the perpendicular bisector, call it P. Using the "distance and length tool"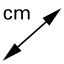 
, measure the distances PA and PB.
What can you observe?
Can you define the perpendicular bisector as a geometrical locus?
, measure the distances PA and PB.
What can you observe?
Can you define the perpendicular bisector as a geometrical locus?Going on ....
Insert another point C and repeat the construction to get the perpendicular bisector of the line segments AC and BC.
Using the "move tool" 
, move one of the vertexs of the triangle ABC.
What do you notice?
Using the "intersect tool", 
, and selecting two perpendicular bisector, you can get their intersection point.
Move again one of the vertexs of the circumference and .....
, move one of the vertexs of the triangle ABC.
What do you notice?
Using the "intersect tool", , and selecting two perpendicular bisector, you can get their intersection point.
Move again one of the vertexs of the circumference and .....
Conclusion
The intersection point of the perpendicular bisectors has an important property. Can you figure it out?
It is ................................. from all the ....................................... of the triangle and, for this reason, it is called ...................................................
So we can say that , given three nocollinear points, it is possible draw the .................................. that passes through all those points.