Exploring Converse of Isosceles Triangle Theorem
- Author:
- Tim Brzezinski
- Topic:
- Angles, Geometry, Isosceles Triangles, Triangles
In the GeoGebra Geometry app window below:
1. Use the ANGLE WITH GIVEN SIZE tool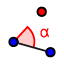 
to construct an angle with side that measures degrees and has a vertex at A. Notice is the name of the slider you see.
2. After doing so, use the RAY tool 
to construct ray AB'.
The silent video show how you can do both of these steps below.
to construct an angle with side that measures degrees and has a vertex at A. Notice is the name of the slider you see.
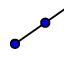2. After doing so, use the RAY tool to construct ray AB'.
The silent video show how you can do both of these steps below. Keep going: More directions appear below this applet.
3. Use the ANGLE WITH GIVEN SIZE tool to construct an angle with side that measures degrees and has a vertex at B. After selecting this tool again, make sure to select A, then B, in that order, and make sure to rotate degrees clockwise.
4. After doing so, use the RAY tool to construct ray BA'.
5. Now use the INTERSECT tool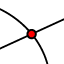 
to plot the point where the two rays (you constructed in steps (2) and (4) intersect. GeoGebra should label this point as C.
6. Right click on each ray and uncheck SHOW OBJECT to hide it. Do the same for B' and A'.
7. Use the POLYGON tool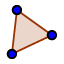 
to construct .
8. Use the DISTANCE or LENGTH tool 
to measure the lengths of segments and .
to construct an angle with side that measures degrees and has a vertex at B. After selecting this tool again, make sure to select A, then B, in that order, and make sure to rotate degrees clockwise.
4. After doing so, use the RAY tool to construct ray BA'.
5. Now use the INTERSECT tool to plot the point where the two rays (you constructed in steps (2) and (4) intersect. GeoGebra should label this point as C.
6. Right click on each ray and uncheck SHOW OBJECT to hide it. Do the same for B' and A'.
7. Use the POLYGON tool to construct .
8. Use the DISTANCE or LENGTH tool to measure the lengths of segments and . What do you notice? (Be sure to select the MOVE tool 
again and move points A and B around!