IM Geometry Unit 2 Lesson 13
Notice and Wonder: Diagonals
What do you notice? What do you wonder?
- Use the tools available to convince yourself the conjecture is true by constructing parallelogram ABCD.
- Convince your partner that the conjecture is true for any parallelogram. Can the 2 of you think of different ways to convince each other?
- What information is needed to prove that the diagonals of a parallelogram bisect each other?
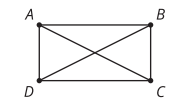
Given: ABCD is a parallelogram with AB parallel to DC and AD parallel to BC. Diagonal AC is congruent to diagonal BD. Prove: ABCD is a rectangle (angles A, B, C, and D are right angles). With your partner, you will work backwards from the statement to the proof until you feel confident that you can prove that is a rectangle using only the given information. Start with this sentence: I would know ABCD is a rectangle if I knew _____________________. Then take turns saying this sentence: I would know [what my partner just said] if I knew __________________. . Write down what you each say. If you get to a statement and get stuck, go back to an earlier statement and try to take a different path.