Teaching Geometry Using Transformations
It has been a couple of years since I have taught geometry, but when I did, my geometry curriculum revolved around the transformation tools: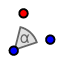 
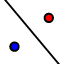
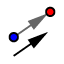
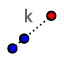and 
.
If my kids needed to construct a perpendicular line, they understood that they would need to perform a 90o rotation.
and .
If my kids needed to construct a perpendicular line, they understood that they would need to perform a 90o rotation.or if they needed to construct a parallel line, they understood that they needed to perform a translation.
Much of my geometry curriculum came from trying to make sense of what these different transformation tools did and did not do.
- What things stayed the same?
- What things changed?
- What happened when you combined these tools?
My kids learned that reflections, rotations, translations were the "big 3" isometries. They learned that reflections were the building blocks of all isometries, and that combining two, or three reflections produced either a rotation, a reflection, or a glide-reflection.
It was only natural that our study of similarity grew out of trying to understand the dilation tool in the same way we understood the rigid transformation tools.
in the same way we understood the rigid transformation tools.All the similarity theorems grew out of our conclusions that we drew regarding the dilation tool.
The first day of the "similarity" unit was really the first day of the "dilation tool" unit. On that day, I created a bunch of "What does this slider do?" types of activities like the ones above and the kids played and reported out what they observed. I created activities that would lay the groundwork for the theorems we would want to establish.