Outline
Complex Numbers
In addition, students working at HL
should be able to
– use the Conjugate Root Theorem to
find the roots of polynomials
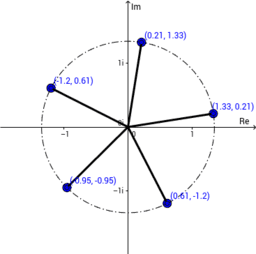
– work with complex numbers in
rectangular and polar form to solve
quadratic and other equations including
those in the form zn = a, where n ∈ Z
and z = r (Cos θ + iSin θ )
– use De Moivre’s Theorem
– prove De Moivre’s Theorem by
induction for n ∈ N
– use applications such as nth roots of
unity, n ∈ N, and identities such as
Cos 3θ = 4 Cos3 θ – 3 Cos θ