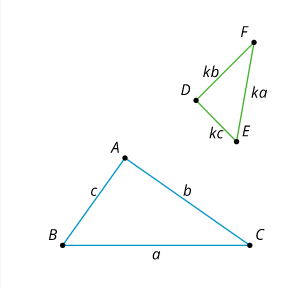
IM Geometry Unit 3 Lesson 10
Evaluate mentally. Is there enough information to determine if the pairs of triangles are congruent? If so, what theorem(s) would you use? If not, what additional piece of information could you use?
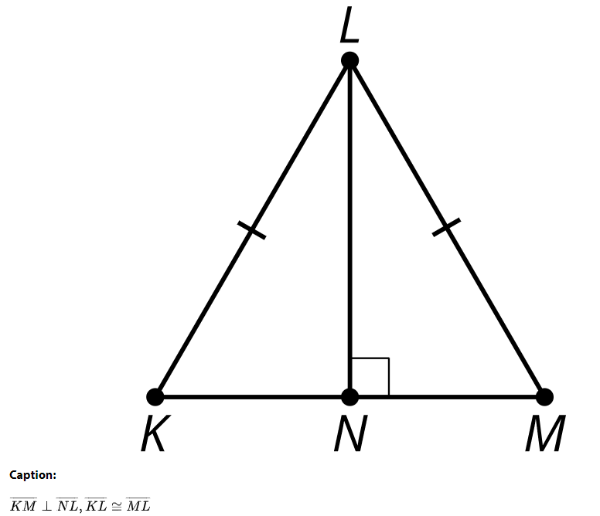
Evaluate mentally. Is there enough information to determine if the pairs of triangles are congruent? If so, what theorem(s) would you use? If not, what additional piece of information could you use?

Evaluate mentally. Is there enough information to determine if the pairs of triangles are congruent? If so, what theorem(s) would you use? If not, what additional piece of information could you use?
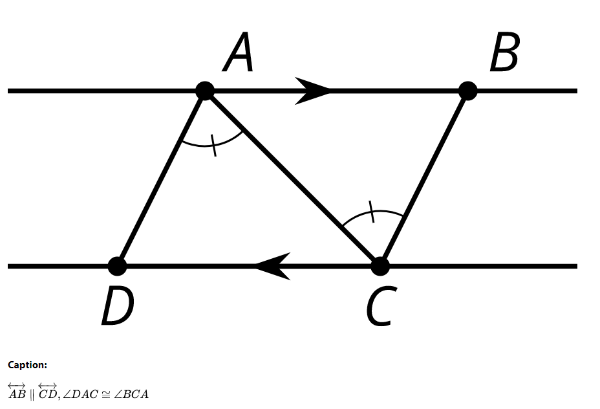
Evaluate mentally. Is there enough information to determine if the pairs of triangles are congruent? If so, what theorem(s) would you use? If not, what additional piece of information could you use?
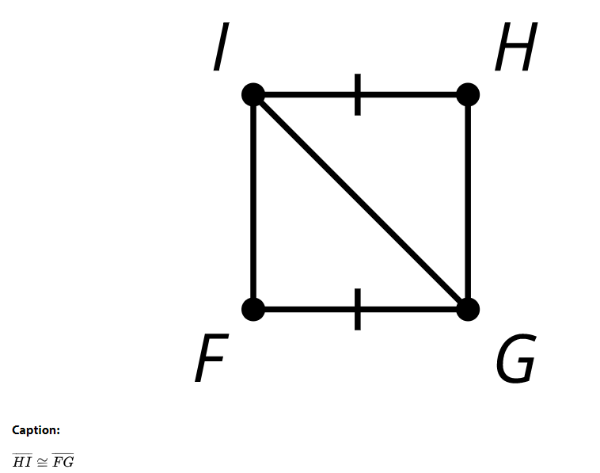
Andre remembers lots of ways to prove triangles congruent. He asks Clare, “Can we use Angle-Side-Angle to prove triangles are similar?”
Clare: “Sure, but we don’t need the Side part because Angle-Angle is enough to prove triangles are similar.”
Andre: “Hmm, what about Side-Angle-Side or Side-Side-Side? What if we don’t know 2 angles?”
Clare: “Oh! I don’t know. Let’s draw a picture and see if we can prove it.”
Andre: “Uh-oh. If ‘side’ means corresponding sides with the same length, then we’ll only get congruent triangles.”
Prove that these 2 triangles must be similar.