Image & Pre-Image of a Functions
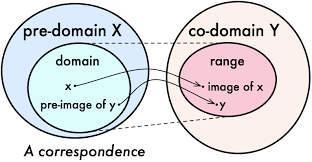
Consider the function f : R → R defined as f (x) = x2 + 3.
Find f [−3,5] and f -1 [12,19].
Answers: f [−3,5] = [12,28];
f -1 [12,19] = [−4,−3]∪[3,4].
Explantion
To find the image of a set under a function and the pre-image of an interval under the inverse of a function, we need to apply the function and its inverse to the given set or interval, respectively.
Given the function f: Z→Z defined as f(x) = x2 + 3, let's find the following:
a)Image of [-3, 5] under f, denoted as f[-3, 5]:
To find the image of the interval [-3, 5] under the function f, we need to apply f(x) to each value in the interval and collect the resulting values.
f(-3) = (-3)2 + 3 = 9 + 3 = 12
f(5) = 52 + 3 = 25 + 3 = 28
The mage of [-3, 5] under f is the interval [12, 28], denoted as f[-3, 5] = [12, 28].
b)Pre-image of [12, 19] under f--1,denoted as f--1[12, 19]:
To find the pre-image of the interval [12, 19] under the inverse function f-1,
we need to apply f--1(x)
to each value in the interval and collect the resulting values. For x in [12, 19],
we want to find f--1(x).
Given that f(x) = x2 + 3, we set x = x2 + 3 and solve for x2: x - 3 = x2
Now, we can use the quadratic formula to find x2:
x2 =
Since we are only interested in the real values of x, we consider the positive and
negative solutions separately:
For x:  [12, 19]:
x2 = and x2 = (to o ensure we have real values).
Solving the inequalities for x, we get: and .
Therefore, the pre-image of [12, 19] under f-1 is the union of the intervals [-4, -3] and [3, 4]. denoted as f--1[12, 19] = [-4, -3] [3, 4].
In summary, for the function f: Z Z defined as f(x) = x2 + 3, we have: f[-3,5]) = [12, 28]
Image of the interval [-3, 5].f--1[12, 19]) = [-4, -3] [3, 4]
Pre-image of the interval [12, 19] under the inverse function f-1.
[12, 19]:
x2 = and x2 = (to o ensure we have real values).
Solving the inequalities for x, we get: and .
Therefore, the pre-image of [12, 19] under f-1 is the union of the intervals [-4, -3] and [3, 4]. denoted as f--1[12, 19] = [-4, -3] [3, 4].
In summary, for the function f: Z Z defined as f(x) = x2 + 3, we have: f[-3,5]) = [12, 28]
Image of the interval [-3, 5].f--1[12, 19]) = [-4, -3] [3, 4]
Pre-image of the interval [12, 19] under the inverse function f-1.
Your Turn.
This problem concerns functions f : {1,2,3,4,5,6,7,8} → {0,1,2,3,4,5,6} How many such functions have the property that |f-1{(2)}| = 4?