Introduction to Poincare and Klein Models

Introduction

As we have learned, one can visualize hyperbolic geometries as a surface in Euclidean Three-Space and manipulate lines, points, and other objects on those surfaces. But, as someone who has interacted with geometry in a purely euclidean way, hyperbolic geometry seems next to impossible to easily explore. This leads to the question: Is it possible to model hyperbolic geometry on a Euclidean plane? This question was answered in the 19th century, with both the Klein Model and Poincaré Model of hyperbolic geometry.

Defining the Models and Distance

Both the Klein and Poincare models use a disk

(a unit circle) to model hyperbolic geometry. All points are contained within the disk, and the boundaries of the disk are ideal endpoints of every line. On the Klein model, lines are defined as open chords of the circle, which is the line segment between two points on boundary of the circle without the endpoints being included. On the Poincare model, lines are defined as either: 1) open diameters of the disk (open chords that go through the center of the disk) or 2) Open circular arcs orthogonal to the disk. On the section below, play around with lines on the Poincare and Klein models.

Drag points P and Q to show different lines on the Klein Model

Drag points P and Q to show different lines on the Poincare Model

What did you notice about the lines on the Klein Model and Poincare Model?

What did you notice about the distance between the two points on the Klein and Poincare model? As one point gets closer to the boundary of the disk, what happens to the distance?

Euclid's 5th Postulate

As the Klein and Poincare Models are modeling hyperbolic geometry, it should be possible to violate Euclid's 5th postulate. Remember that a statement equivalent to Euclid's 5th postulate is "Given a line and a point not on the line, at most one line parallel to the given line can be drawn through the point." (Playfair's posulate) Use the figures below to show that Euclid's 5th postulate does not hold on the Klein or Poincare Models

Drag points to show Euclid's 5th postulate does not hold on the Klein Model

Drag points and change the radius of a circle on the tangent lines at those points to show Euclid's 5th postulate does not hold on the Poincare Model

Were you able to show that Euclid's 5th Postulate does not hold on the Klein and Poincare Models? Why or why not?

Angle Measures

Now, let's take a look at one of the largest problems with the Klein model. Angles. Perpendicular lines on the Klein model are constructed using the Pole of a chord C. Any chord that, when extended into a line, passes through the pole of C is perpendicular to C. Play around with the model below. Both open BC and AD are perpendicular to AB.

Drag points A and B to explore angles on the Klein Model

What do you notice about the perpendicular angles CBA and DAB? Do they always look congruent?

Angle measure is complicated on the Klein Model. Angles that look congruent aren't always congruent, and angles that look incongruent could be congruent. This is a problem that the Poincare Model does not have. On the Poincare disk, angles are measured in the same way as in Euclidean geometry. Because of this, the easiest way to measure angles on the Klein Model is to translate the points to the Poincare Model, and then measure the angle in that model. To do this, we need a way to translate the Klein Model to the Poincare Model (and vice versa).

Relating the Poincare and Klein Models

It is the case that the two models can be related via an isomorphism (a one to one and onto function which maps points and lines from one model to the other while preserving betweenness and congruence). One such isomorphism is given as follows. Let S be the unit sphere in

Let

be the unit circle on the xy plane.

represents both the Klein and Poincare model. To start, project every point in the Poincare Model onto the southern hemisphere of S stereographically from the north pole of S (i.e. construct a line from the north pole through a point P on the Poincare model. The intersection point P' of line NP and the southern hemisphere of S is the steriographic projection of P from the north pole of S.) Now, project orthogonal upward to the disk . (i.e. construct a line through P' orthogonal to the disk. The point P'' where the line intersects the disk is the orthogonal projection of P'. Note that because the disk is contained in the xy-plane, if P' = (a, b, c), then P'' = (a, b, 0.) This process maps every point on the Poincare Model to every point on the Klein model (while preserving lines, congruence, and betweenness). Take a look at the model below to see how points are mapped from the Poincare to Klein model

Drag around the point P on the Poincare Disk to see where it's image on the Klein Disk (K) is mapped to.

This process can be represented by a function F where

This allows us to easily map from the Poincare Disk to the Klein Disk (or vice versa). Explore the model below to see how lines are related between the two models.

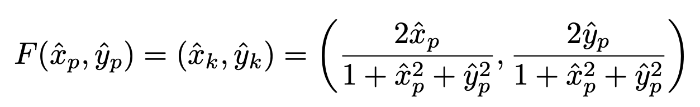
Drag points P and Q on the Poincare Model to see their images on the Klein Model and the lines produced on each model.

What do you notice about the relationship between the same line on the Poincare and Klein models? When does a line on the Poincare Disk and its image on the Klein Disk overlap? When does a point on the Poincare Disk and its image on the Klein Disk overlap?

Using the Poincare Model to discover facts about hyperbolic geometry

Let's use the Poincare Model to try and discover facts about triangles in hyperbolic geometry.

Drag points P Q and R to explore triangles on the Poincare Model

What did you discover?

What is the largest triangle angle sum you can achieve on a Poincare Disk?

What's the smallest triangle angle sum you can achieve on a Poincare Disk? (Note: points on the edge of the disk are not points in Hyperbolic geometry, but are instead ideal points at infinity.)

Quadrilaterals on a Poincare Disk

Finally, let's take a look at quadrilaterals on a Poincare Disk. Take some time to explore and discover as much as you can.