Introduction
Have you ever watched a basketball arcing through the air into the hoop, or noticed how a fountain's water makes a curved path as it falls back to the ground? What you're observing is a beautiful example of projectile motion - a fundamental concept that connects mathematics, physics, and engineering.
Projectile motion describes the movement of an object thrown or projected into the air, subject to external forces (e.g.). This motion is predictable and follows a specific path called a trajectory. The trajectory of a simple projectile is a parabola – a shape you might recognize from your math classes.
Let's throw an object with initial velocity initial height , and angle of elevation .
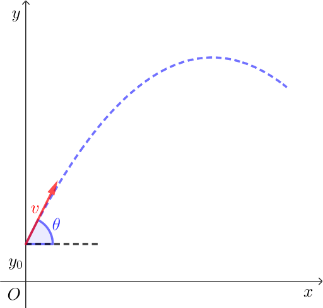
The height metres of the ball whose horizontal distance is metres away is given by the equation
where , , and .
For this worksheet, we will fix . We will therefore use the simplified equation to model the projectile motion:
where denotes the angle of elevation in degrees, denotes the initial height, and denotes the gravitational constant on Earth.