L1.10 - Rigid Transformations
Learning Intentions and Success Criteria
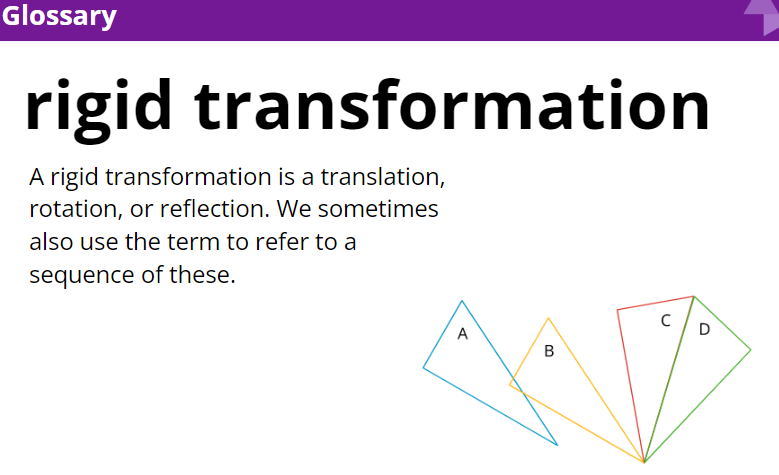
- Comprehend that rigid transformations produce congruent figures by preserving distance and angles.
- Draw the result of a transformation (in written language) of a given figure.
- Explain (orally and in writing) a sequence of transformations to take a given figure onto another.
- Draw the figure's image after the transformation when given a figure and the description of a transformation.
- Describe the sequence of transformations necessary to take a figure onto another figure.
- Know that rigid transformations result in congruent figures.
10.1: Notice and Wonder: Transformed
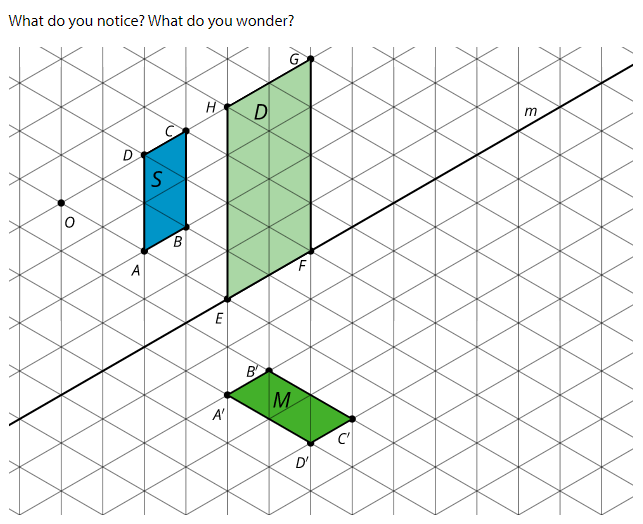
What do you notice? What do you wonder?

10.2: What’s the Same?
Draw each rigid transformation. Use the Style Bar to choose a different color for each one.
1. Translate figure S along the line segment v in the direction shown by the arrow. Color:
2. Reflect figure S across line n. Color:
3. Reflect figure S across line m. Color:
4. Translate figure S along the line segment w in the direction shown by the arrow. Reflect this image across line n. Color:
5. How are the images the same? How are they different?
10.3: Does Order Matter?
1. Describe a sequence of transformations that will take Figure A onto Figure B.
2. If you reverse the order of your sequence, will the reverse sequence still take A onto B?
3. Describe a sequence of transformations that will take Figure A onto Figure C.
4. If you reverse the order of your sequence, will the reverse sequence still take A onto C?
Learning Intentions and Success Criteria
- Comprehend that rigid transformations produce congruent figures by preserving distance and angles.
- Draw the result of a transformation (in written language) of a given figure.
- Explain (orally and in writing) a sequence of transformations to take a given figure onto another.
- Draw the figure's image after the transformation when given a figure and the description of a transformation.
- Describe the sequence of transformations necessary to take a figure onto another figure.
- Know that rigid transformations result in congruent figures.
Cool-Down: How Will That Get There?