Properties of Tangents Drawn to Circles (VC)
DIRECTIONS:
In the applet below, note the circle with center A.
You can alter the radius by dragging the unlabeled point on the circle.
Point B is a point on the circle.
Point C is a point that lies outside the circle.
Feel free to alter the locations of any of these 4 points at any time.
1. Select the TANGENTS 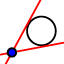 tool. Then select point B and then select the circle. This will construct a line through B that is tangent to the circle.
2. Use the SEGMENT
tool. Then select point B and then select the circle. This will construct a line through B that is tangent to the circle.
2. Use the SEGMENT 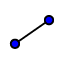 tool to construct radius .
3. Use the POINT ON OBJECT
tool to construct radius .
3. Use the POINT ON OBJECT 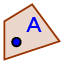 tool to plot a point D on this tangent line.
Use the ANGLE
tool to plot a point D on this tangent line.
Use the ANGLE 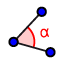 tool to measure . (Be sure to select the points in this order.)
tool to measure . (Be sure to select the points in this order.)
 tool. Then select point B and then select the circle. This will construct a line through B that is tangent to the circle.
2. Use the SEGMENT
tool. Then select point B and then select the circle. This will construct a line through B that is tangent to the circle.
2. Use the SEGMENT  tool to construct radius .
3. Use the POINT ON OBJECT
tool to construct radius .
3. Use the POINT ON OBJECT  tool to plot a point D on this tangent line.
Use the ANGLE
tool to plot a point D on this tangent line.
Use the ANGLE  tool to measure . (Be sure to select the points in this order.)
tool to measure . (Be sure to select the points in this order.) 4. Select the MOVE  tool. Move point B around the circle. What do you notice?
tool. Move point B around the circle. What do you notice?
5. Use the TANGENTS  tool to construct a line passing through C that is tangent to the circle.
How many tangents can be drawn from point C to the circle?
tool to construct a line passing through C that is tangent to the circle.
How many tangents can be drawn from point C to the circle?
6. Use the INTERSECT  tool to plot the point(s) at which these tangents intersect the circle.
GeoGebra should name these points E and F.
tool to plot the point(s) at which these tangents intersect the circle.
GeoGebra should name these points E and F.
 tool to plot the point(s) at which these tangents intersect the circle.
GeoGebra should name these points E and F.
tool to plot the point(s) at which these tangents intersect the circle.
GeoGebra should name these points E and F. 7. Use the DISTANCE OR LENGTH  tool to measure the distance from C to E and the distance from C to F. What do you notice?
tool to measure the distance from C to E and the distance from C to F. What do you notice?
8. What two new properties can we conclude about tangents drawn to circles? Describe.