Archimedes' Spiral
Propositions 18 and 19I. If OA be the initial line, A the end of the first turn of the spiral, and if the tangent to the spiral at A be drawn, the straight line OB drawn from ) perpendicular to OA will meet the said tangent in some point B, and OB will be equal to the circumference of the "first circle".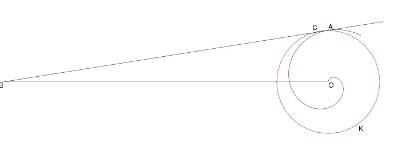 
Proof: Suppose OB is greater than c, the circumference of the first circle.
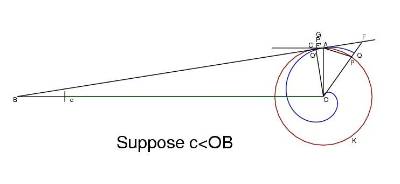Suppose OB is less than c, the circumference of the first circle.
Since OB is not less than or greater than c, OB = c.
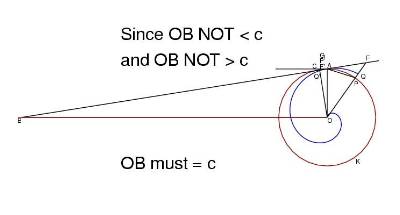II. If A' be the end of the second turn, the perpendicular OB will meet the tangent at A' in some point B', and OB' will be equal to 2(circumference of the "second circle").
Proof: Suppose OB is greater than 2c', twice the circumference of the second circle.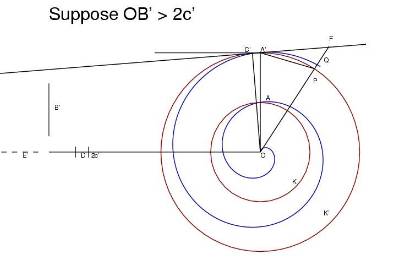
Suppose OB is less than 2c', twice the circumference of the second circle.
Since OB is not less than or greater than 2c', OB = 2c'.
III. Generally, if An be the end of the nth turn, and OB meet the tangent at An in Bn, then
OBn = ncn,
where cn is the circumference of the "nth circle"
The proof for this is similar to the proofs for the first and second circles.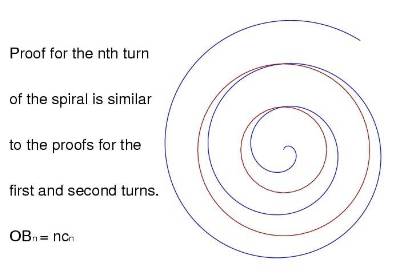
Proof: Suppose OB is greater than c, the circumference of the first circle.
Suppose OB is less than c, the circumference of the first circle.
Since OB is not less than or greater than c, OB = c.
II. If A' be the end of the second turn, the perpendicular OB will meet the tangent at A' in some point B', and OB' will be equal to 2(circumference of the "second circle").
Proof: Suppose OB is greater than 2c', twice the circumference of the second circle.
Suppose OB is less than 2c', twice the circumference of the second circle.
Since OB is not less than or greater than 2c', OB = 2c'.
III. Generally, if An be the end of the nth turn, and OB meet the tangent at An in Bn, then
OBn = ncn,
where cn is the circumference of the "nth circle"
The proof for this is similar to the proofs for the first and second circles.