L6.5 - Squares and Circles
Learning Intentions and Success Criteria
- Calculate and interpret trinomials in expanded and factored form in equations for circles.
- Understand how squared binomials relate to the equation of a circle.
5.1: Math Talk: Distribution
- 5(x + 3)
- x(x - 3)
- (x + 4)(x + 2)
- (x - 5)(x - 5)
5.2: Perfectly Square
1. Apply the distributive property to each expression. a. (x - 7)(x - 7)
b. (x + 4)2
c. (x - 10)2
d. (x + 1)2
2. Look at your results. Each of these expressions is called a perfect square trinomial. Why?
3 & 4. Which of these expressions are perfect square trinomials? If you get stuck, look for patterns in your earlier work. Rewrite the perfect square trinomials you identified as squared binomials. a. x2 - 6x + 9
b. x2 + 10x + 20
c. x2 + 18x + 81
d. x2 - 2x + 1
e. x2 + 4x + 16
5.3: Back and Forth
1. Here is the equation of a circle: (x - 2)2 + (y + 7)2 = 102 a. What are the center and radius of the circle?
b. Apply the distributive property to the squared binomials and rearrange the equation so that one side is 0. This is the form in which many circle equations are written.
2. This equation looks different, but also represents a circle: x2 + 6x + 9 + y2 – 10y + 25 = 64 a. How can you rewrite this equation to find the center and radius of the circle?
b. What are the center and radius of the circle?
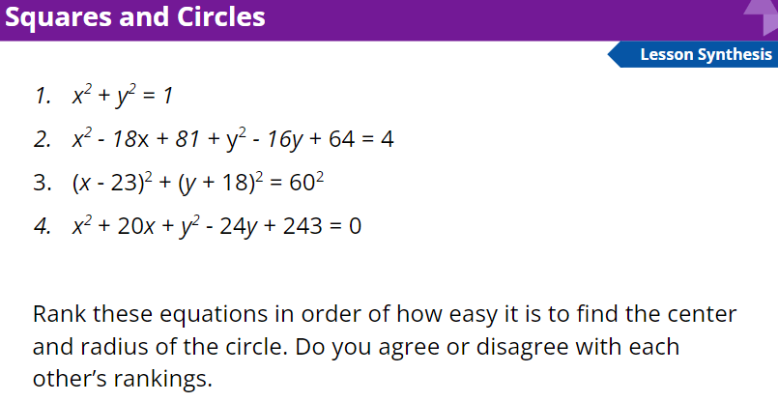

Learning Intentions and Success Criteria
- Calculate and interpret trinomials in expanded and factored form in equations for circles.
- Understand how squared binomials relate to the equation of a circle.
Cool-Down: Center and Radius
Find the center and radius of the circle with this equation: x2 + 16x + 64 + y2 - 2y + 1 = 144