Extending the problem of poles and strings
Instructions.
1) Use the slider to change the distance 'b' between C and D.
2) Move the blue point B to change the distance 'a' between A and B.
3) Move the point C to change the distance between the points A and C.
4) Move the point D to change the base angles.
Observe the changes that happen while you move the large blue points and the slider.
1) Does the value of 'y' change as you change (only) the distance between the point A and C.
2) Does the value of 'y' depend on both the values 'a' and 'b'?
3) By changing the base angle is there a change in the value 'y'?
4) What are factors that determine the value of 'y'?
Deriving the formula for calculating y.
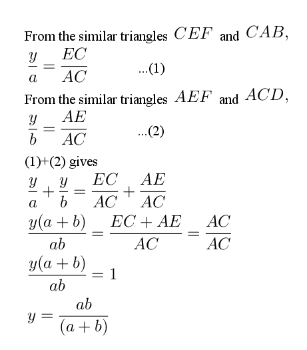
Problem of Poles and Strings.
There are two vertical poles some distance apart. Strings are tied (tightly streched) from the top of each pole to the base of the other pole. We are to calculate the height (perpendicular) of the point where the strings meet from the ground.
Bhāskarāchārya ("Bhāskara, the teacher"), popularly known as Bhaskara II, in his work Lilāvatī, gives the rule to calculate the height (perpendicular) of the meeting point of the ropes from the ground and the distance of the perpendicular from each of the two poles on the ground.
अन्योन्यमूलाग्रगसूत्रयोगात्
वेण्योर्वधे योगहृते च लंबः।
वंशौ स्वयोगेन हृतौ अभीष्ट-
भूघ्नौ च लंबोभयतः कुखंडे॥ CLXVII
; and