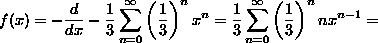Maclaurin Series
Power Series Reflection
The Maclaurin series is a template that allows you to express many other functions as power series. It is the source of formulas for expressing both sin x and cos x as infinite series.Without further ado, here it is:
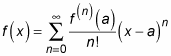The notation f(n) means “the nth derivative of f.” This becomes clearer in the expanded version of the Maclaurin series:
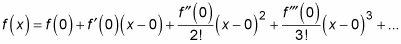The Maclaurin series allows you to express functions as power series by following these steps:
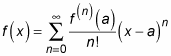The notation f(n) means “the nth derivative of f.” This becomes clearer in the expanded version of the Maclaurin series:
The Maclaurin series allows you to express functions as power series by following these steps:- Find the first few derivatives of the function until you recognize a pattern.
- Substitute 0 for x into each of these derivatives.
- Plug these values, term by term, into the formula for the Maclaurin series.
- If possible, express the series in sigma notation.
For instance, suppose you were interested in finding the power series representation of
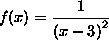We can find the power representation of this function like so:
,
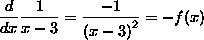so
r
.
,
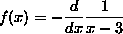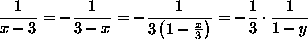where y=x/3, so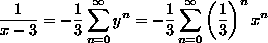 
.
Thus,
We can find the power representation of this function like so:
,
so
r.,
where y=x/3, so .
Thus,