Fractal curves - points and edges
One possible solution
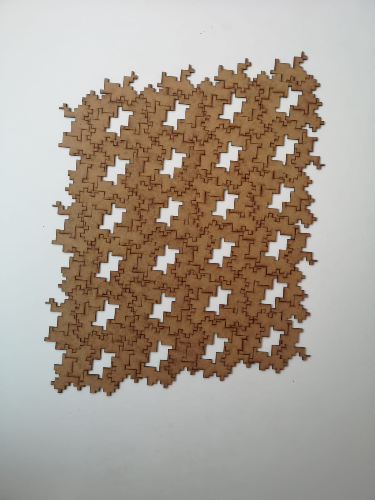
Fractal game workshop plan
Materials:
puzzle-parts (each pupil gets a package à 55 pieces)
possibly a beamer for presenting the photos of the puzzles
Learning outcomes:
Recognising repetitions and symmetries, sharpening perception of the environment, recognising that sequences and series are also a way of repetition, learning mathematical descriptions of repetitions through fractals, patterns, enlargements and reductions, ...
Curricula:
- primary school (VS, 6-10 years): symmetries, perception, surfaces, shapes, ...
- lower secondary school (Sek I, 10-14 years): (Self-)similarity, uniform scaling
- upper secondary school (Sek II, 14-18 years): fractals, sequences and series
- teacher-centered instruction (frontal)
- individual work
- plenary
- group work

Introduction
- teaching method: frontal
- duration: 10 min + 5 min time buffer
- materials: photos, pictures, videos, vegetables, pine cones, ...
- consider if you include the video!?
- students should bring objects such as pine cones, ...
- pass objects through, let them discover regularities and repetitions
- show video, show pictures of objects that contain repetitions
- show scaling using GeoGebra
- show video, show pictures of objects that contain repetition
- explaining mathematical concepts of sequences and series
- explaining the meaning of endless repetition, Mandelbrot set, ...
Puzzling alone - find your personal solution
- teaching method: individual work
- duration: 20 min + 10 min
- material: puzzle
Reflection - what did you like about your solution?
- teaching methods: plenary discussion
- duration: 15 Minuten
- material: finished puzzle-results, beamer (for presenting photos of further puzzle-results)
Connecting puzzles
- teaching method: group work
- duration: 30 min
- material: puzzle
Follow up after the workshop
primary school
"Geometric forms arise from different (other) geometric forms", no longer just "the round must go into the round".
lower secondary school
students should work with applets of e.g. Diego Lieban: cutting up triangles, ... so that the result is self-similar.
upper secondary school
continue with the topics "sequences and series"