Angle Bisector Proportionality - 2019 (Unit 5 lesson 5)
Follow the instructions on the diagram below
Step 1: Click this angle bisector icon 
and then click the points A, B and C in that order to draw the angle bisector for ABC.
Step 2: Click this intersection icon 
and then choose the angle bisector line and AC. You should see a new point at the intersection.
Step 3: To label the new point, click the label icon 
and the new point. It should say D. If not, restart and redo steps 1-3.
Step 4: Measure the bisected angle using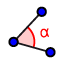 
and clicking on 3 points making sure that the vertex is the middle one.
Step 5: Using the 
drag around point C on the triangle to make sure that the line you drew always bisects the angle into two equal halves.
Step 6: Have your teacher check your work before moving to the next section.Angle Bisector Proportionality Theorem
On the same diagram, add the following measurements.
Step 7: Click on segment measurement icon 
and the points A and B to show the length of AB.
Step 8: Use 
to also show the lengths of BC, AD and DC.
and the points A and B to show the length of AB.
Step 8: Use to also show the lengths of BC, AD and DC.
Comparison of lengths.
Use 
to move point C so that AB = BC. What do you notice about AD and DC?
Comparison of lengths.
Now move point C so that AB is longer than BC. What do you notice about AD and DC? Now move point C so that AB is shorter than BC. In the space below, describe what you noticed.
Comparison of lengths.
Now move point C so that BC is the same length as DC. What do you notice about BC and DC?
Angle Bisector Proportionality Theorem
On the sketch above, click on buttons to see side lengths and ratios. As you drag the vertices of the triangle, pay attention to which ratios stay equal.
Which of the following is not a ratio that is always equal?
Find x in the diagram below. It shows an angle bisector but is not drawn to scale.
Use this applet to discover the angle bisector proportionality theorem!
What is the value of x?
